A branch of mathematics that deals with the collection, organization, analysis, interpretation, and presentation of data is known as statistics. As far as any entrance exam is concerned, students can expect 1-2 questions from statistics. Descriptive statistics and inferential statistics are the two major areas of statistics. The branch which describes the properties of sample and population data is called descriptive statistics. The branch of statistics which uses those properties to test hypotheses and draw conclusions are called inferential statistics.
The mathematical theories behind statistics depend on probability theory, differential and integral calculus and linear algebra. An important theorem in statistics is the central limit theorem (CLT). According to this theorem, for any population having mean and standard deviation, the distribution of the sample mean for sample size N has mean μ and standard deviation σ / √n. We can also state that when the large sample size is having a finite variance, the samples will be normally distributed. The mean of samples will be almost equal to the mean of the whole population.
Assumptions of Central Limit Theorem
Following are the assumptions of CLT.
- The size of the sample should be adequately large.
- The sample should be randomly drawn by satisfying the condition of randomization.
- These should be independent of each other and should not impact the other samples.
- When the sampling is done without replacement, the sample size shouldn’t exceed ten percent of the total population.
Applications of Central Limit Theorem
Let us have a look at some applications of CLT.
1) CLT can be used to tell whether the sample belongs to a particular population by looking at the sample distribution.
2) This theorem is used in finding the mean family income in a particular country.
3) This is used in election polls to estimate the percentage of people supporting a particular candidate as confidence intervals.
4) It enables us to make conclusions about the sample and population parameters and assists in constructing good machine learning models.
5) According to CLT, when the distribution is unknown or not normally distributed, the sample distribution is considered to be normal. This method concludes that the given population is distributed normally. It also helps in analysis of data.
6) When the samples taken from the population increases, the sample mean deviation decreases which helps in estimating the mean of the population more accurately.
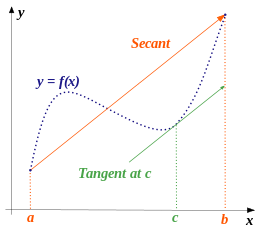
Mean Value Theorem
This theorem helps us to understand the identical behavior of different functions. Mean value theorem (MVT) is used in both the differential and integral calculus. It has very important outcomes in differential calculus. The statement of this theorem is as follows.
Let f(x) is a function that satisfies the conditions: f(x) is continuous in interval [a, b] and f(x) is differentiable in (a, b). Then, there exists a number c, such that a < c < b and f(b) – f(a) =
f ‘(c) (b-a).
The hypothesis and conclusion of this theorem shows some similarities to those of Intermediate value theorem. Visit BYJU’S to learn more about CLT and other related theorems.